I am hosting the analysis seminar for the 2025 spring semester.
Talks will be given in Little Hall 423 on Fridays during period 6 (12:50-1:40 pm).
Here is the list of speaker:
| Speaker | Date | Topic | Topic Description | |
|---|---|---|---|---|
| Arya Memana | 14 February | Free Probability and the Central Limit Theorem | In this talk, we will introduce fundamental concepts in Free Probability Theory. We will also present a combinatorial proof sketch of the classical and free central limit theorems. | |
| David Maynoldi | 21 February | Testing Von-Neumann's Inequality on Matrices | This talk is based on a new paper which gives an alternative proof of a known method for testing Von-Neumann's Inequality. The main result says that instead of testing the inequality on all tuples of commuting contractive operators on a Hilbert space, we can just test on tuples of commuting contractive nilpotent matrices. We will discuss how Caratheodory interpolation and cone separation is used to prove this result. We will state analogous results for testing on tuples of simultaneously diagonalizable contractive matrices. | |
| Lodewyk Jansen van Rensburg | 28 February | On the Connection Between Multipliers of the Drury-Averson Space and Non-commutative Hardy Space via Realizations. | The Drury-Arveson space and Non-Commutative Hardy space arise naturally in operator theory, function theory, and nc-function theory. In this talk, we explore the connections between their multiplier algebras, focusing on the transition between the commutative and non-commutative setting. A key tool in this passage is the use of realizations, which allows us to characterize their closed unit balls. We will discuss how these techniques allow us to bridge the two settings and mention successful application to characterizing extreme points. | |
| Dr. Michael Hartz | 7 March | Von Neumann's inequality on the polydisc | The classical von Neumann inequality shows that for any contraction T on a Hilbert space, the operator norm of p(T) satisfies 
Whereas Ando extended this inequality to pairs of commuting contractions, the corresponding statement for triples of commuting contractions is false. However, it is still not known whether von Neumann's inequality for triples of commuting contractions holds up to a constant. I will talk about this question and about the function theoretic upper bounds for ||p(T)||. | |
| Dr. Woongbae Park | 14 March | Introduction to Regularized n-Conformal Heat Flow |  | |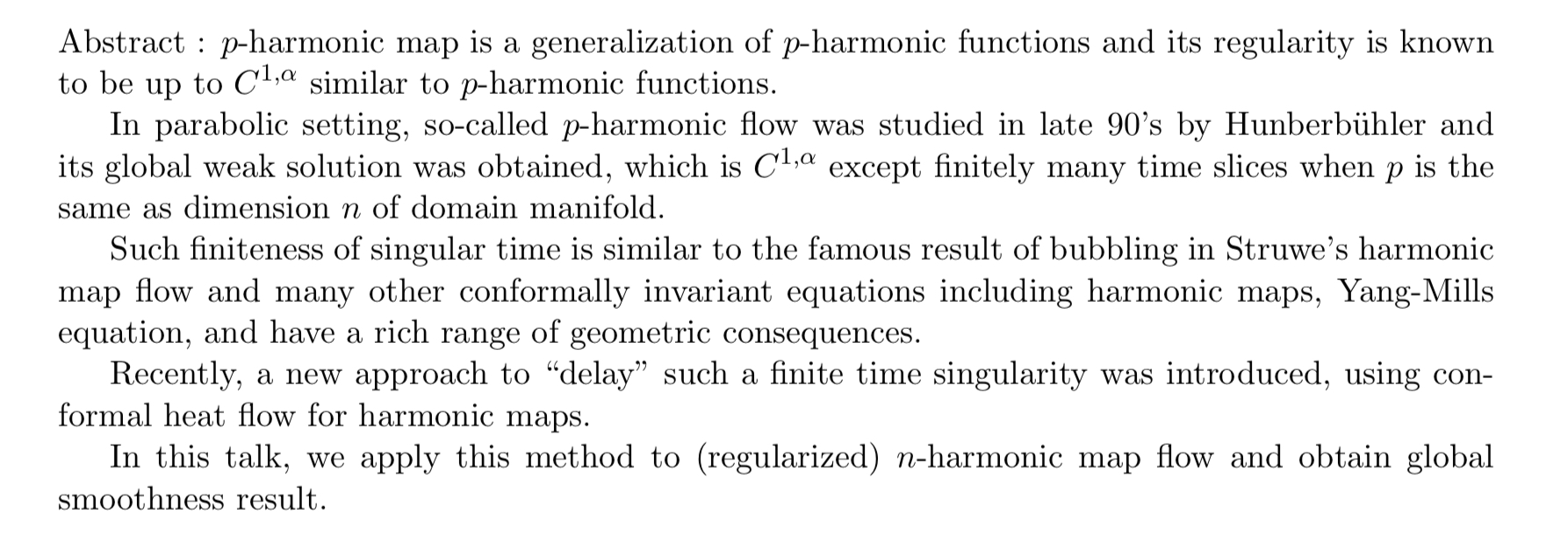
| Vangmay Jayant | 28 March | Every Complete Pick Space Satisfies the Column-Row Property | In the theory of Complete Pick spaces, the column-row property has appeared in a variety of contexts. We discuss a result by Dr. Michael Hartz that shows that it is satisfied by every complete Pick space. In combination with known results, this yields several consequences to the theory of Weak Product spaces, to de Branges-Rovnyak spaces and more. We will discuss an outline of the proof of the result, which uses a variant of the Schur algorithm. | |
| Pranav Chinmay | 4 April | Robust Construction of the Incipient Infinite Cluster in High-Dimensional Percolation | The incipient infinite cluster was first proposed by physicists in the 1970's as a canonical example of a two-dimensional medium on which random walk is subdiffusive. It is the measure obtained in critical percolation by conditioning on the existence of an infinite cluster, which is a probability zero event. Kesten presented the first rigorous two-dimensional construction of this object as a weak limit of the one-arm event. In high dimensions, van der Hofstad and Jarai constructed the IIC as a weak limit of the two-point connection using the lace expansion. Our work presents a new high-dimensional construction which is "robust," establishing that the weak limit is independent of the choice of conditioning. The main tools used are Kesten's original two-dimensional construction combined with Kozma and Nachmias' regularity method. Our robustness allows for several applications, such as the explicit computation of the limiting distribution of the chemical distance, which forms the content of our upcoming project. This is joint work with Shirshendu Chatterjee, Jack Hanson, and Philippe Sosoe. The preprint can be found at https://arxiv.org/abs/2502.10882. | |
| Jacob Levenson | 9 April | Factorization of Polynomials with Operator Coefficients | In this talk, we discuss known results about factoring polynomials whose coefficients are operators on a Hilbert space. After examining polynomials in all commuting variables and polynomials in all n.c. variables, we present a new result about factoring positive polynomials in three variables, only one of which commutes with the other two. | |
| Dr. Eric Evert | 11 April | Inclusion Constants for Matrix Convex sets relevant to Quantum Incompatibility |  | |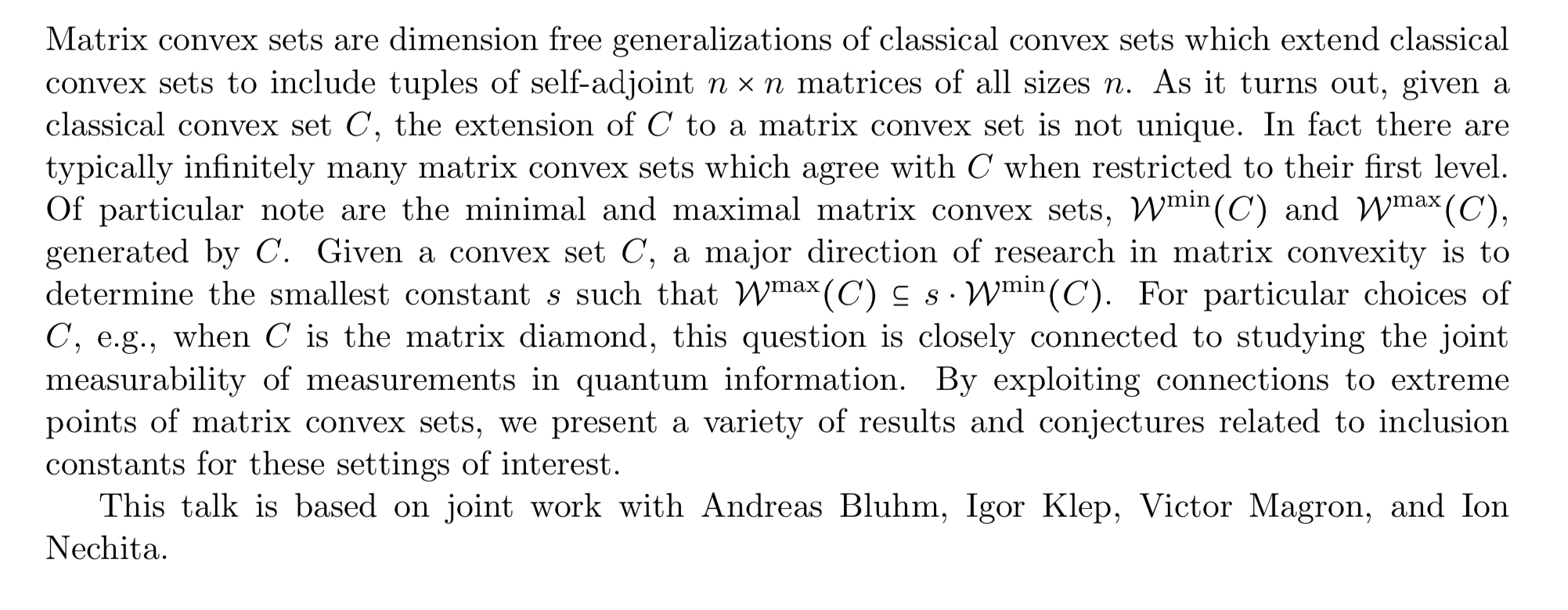
| Dr. Michael Jury | 18 April | |||